Hexagram
| Regular hexagram | |
|---|---|
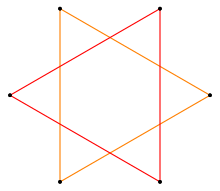
A regular hexagram
|
|
| Type | Regular polygonal figure |
| Edges and vertices | 6 |
| Schläfli symbol | a{6}, {6/2}, 2{3} or {{3}} |
| Coxeter diagram |
|
| Symmetry group | Dihedral (D6) |
| Internal angle (degrees) | 60° |
| Dual polygon | self |
| Properties | star, compound, cyclic, equilateral, isogonal, isotoxal |
A hexagram (Greek) or sexagram (Latin) is a six-pointed geometric star figure with the Schläfli symbol {6/2}, 2{3}, or {{3}}. It is the compound of two equilateral triangles. The intersection is a regular hexagon.
It is used in historical, religious and cultural contexts, for example in Hanafism,Jewish identity, Hinduism and occultism.
In mathematics, the root system for the simple Lie group G2 is in the form of a hexagram, with 6 long roots and 6 short roots.
A six-pointed star, like a regular hexagon, can be created using a compass and a straight edge:
It is possible that as a simple geometric shape, like for example the triangle, circle, or square, the hexagram has been created by various peoples with no connection to one another.
The hexagram is a mandala symbol called satkona yantra or sadkona yantra found on ancient South Indian Hindu temples. It symbolizes the nara-narayana, or perfect meditative state of balance achieved between Man and God, and if maintained, results in "moksha," or "nirvana" (release from the bounds of the earthly world and its material trappings).
...
Wikipedia
