Frustum

Set of pyramidal frustums
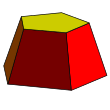
Examples: Pentagonal and square frustum
Faces	n trapezoids, 2 n-gons
Edges	3n
Vertices	2n
Symmetry group	Cnv, [1,n], (*nn)
Properties	convex

In geometry, a frustum (plural: frusta or frustums) is the portion of a solid (normally a cone or pyramid) that lies between one or two parallel planes cutting it. A right frustum is a parallel truncation of a right pyramid.

The term is commonly used in computer graphics to describe the viewing frustum, the three-dimensional region which is visible on the screen. It is formed by a clipped pyramid; in particular, frustum culling is a method of hidden surface determination.

In the aerospace industry, frustum is the common term for the fairing between two stages of a multistage rocket (such as the Saturn V), which is shaped like a truncated cone.

Each plane section is a floor or base of the frustum. Its axis if any, is that of the original cone or pyramid. A frustum is circular if it has circular bases; it is right if the axis is perpendicular to both bases, and oblique otherwise.

The height of a frustum is the perpendicular distance between the planes of the two bases.

Cones and pyramids can be viewed as degenerate cases of frusta, where one of the cutting planes passes through the apex (so that the corresponding base reduces to a point). The pyramidal frusta are a subclass of the prismatoids.

Two frusta joined at their bases make a bifrustum.