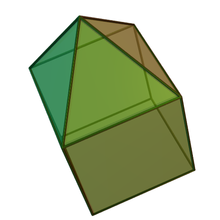
Elongated square pyramid
| Elongated square pyramid | |
|---|---|
 |
|
| Type |
Johnson J7 - J8 - J9 |
| Faces | 4 triangles 1+4 squares |
| Edges | 16 |
| Vertices | 9 |
| Vertex configuration | 4(43) 1(34) 4(32.42) |
| Symmetry group | C4v, [4], (*44) |
| Rotation group | C4, [4]+, (44) |
| Dual polyhedron | self |
| Properties | convex |
| Net | |
 |
|
In geometry, the elongated square pyramid is one of the Johnson solids (J8). As the name suggests, it can be constructed by elongating a square pyramid (J1) by attaching a cube to its square base. Like any elongated pyramid, it is topologically (but not geometrically) self-dual.
A Johnson solid is one of 92 strictly convex polyhedra that have regular faces but are not uniform (that is, they are not Platonic solids, Archimedean solids, prisms or antiprisms). They were named by Norman Johnson, who first listed these polyhedra in 1966.
The dual of the elongated square pyramid has 9 faces: 4 triangular, 1 square and 4 trapezoidal.
The elongated square pyramid can form a tessellation of space with tetrahedra, similar to a modified tetrahedral-octahedral honeycomb.
...
Wikipedia
